Quantum many-body observables in equilibrium are continuous at exceptional points
Published:
TLDR: The exceptional point serves as a non-isolated singularity (branch point) for biorthogonal wavefunctions but as a removable singularity for physical observables.1
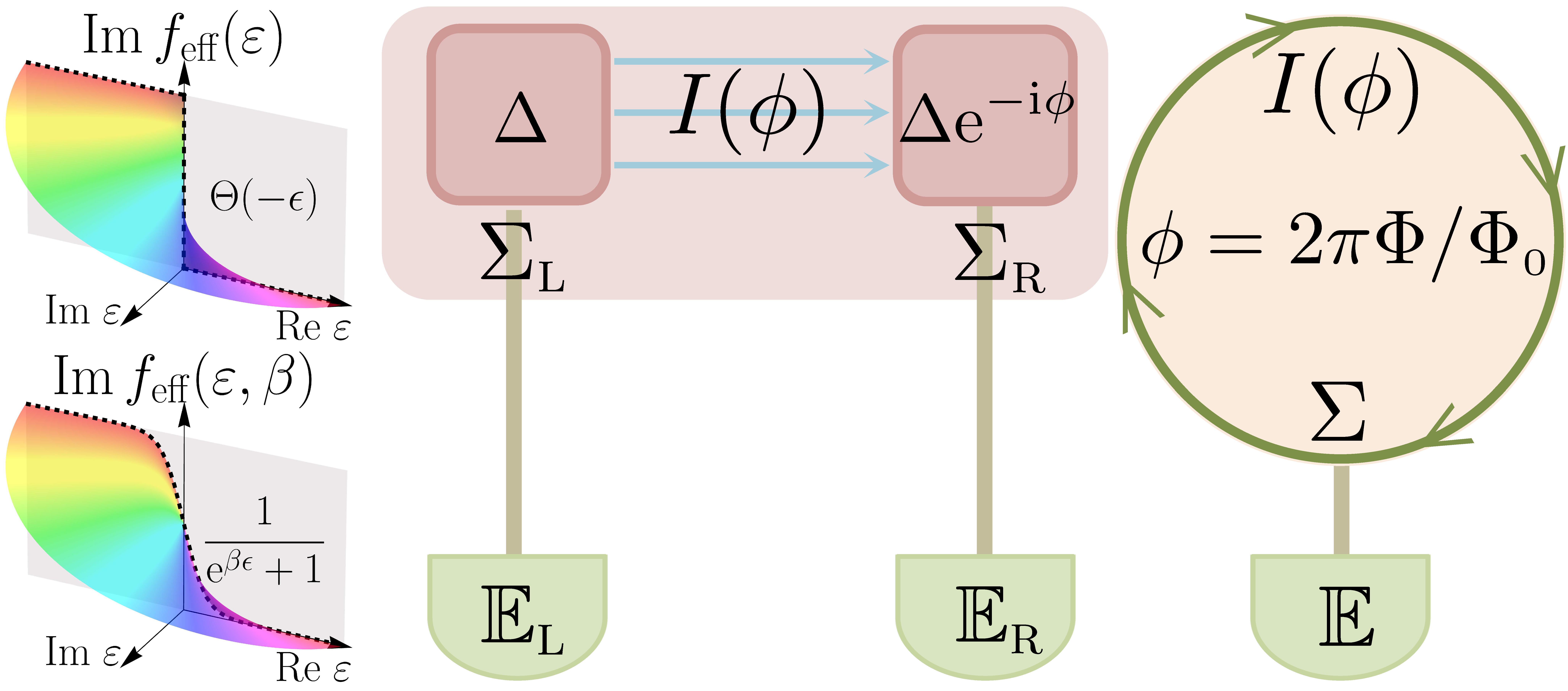
Here I present a simple toy model to explicitly show that exceptional points of a non-Hermitian Hamiltonian do not give divergent behavior for quantum many-body observables in equilibrium.
We consider a toy model with a $2 \times 2$ Hamiltonian ($\kappa>0$):
\[\begin{align*} \mathcal{H}_\mathrm{eff} &= \begin{bmatrix} 0 & 1 \\ 1 & -2 \mathrm{i} \kappa \\ \end{bmatrix} = \sum_{n = \pm} \varepsilon_{n} \ket{\psi^\mathrm{R}_n} \bra{\psi^\mathrm{L}_n} \\ &= \frac{1}{\varepsilon_+ - \varepsilon_-} \begin{bmatrix} +\varepsilon_- & +\varepsilon_+ \\ -1 & -1 \\ \end{bmatrix} \begin{bmatrix} \varepsilon_+ & 0 \\ 0 & \varepsilon_- \\ \end{bmatrix} \begin{bmatrix} -1 & -\varepsilon_+ \\ +1 & +\varepsilon_- \\ \end{bmatrix} \,, \end{align*}\]whose exact eigenvalues $\varepsilon_\pm = - \mathrm{i} \kappa \pm \sqrt{1-\kappa^2}$ and exact biorthogonal wavefunctions are
\[\begin{align*} \begin{bmatrix} \ket{\psi^\mathrm{R}_+} & \ket{\psi^\mathrm{R}_-} \end{bmatrix} = \frac{1}{\sqrt{2}} \begin{bmatrix} +\varepsilon_- & +\varepsilon_+ \\ -1 & -1 \\ \end{bmatrix} \,, \quad \begin{bmatrix} \bra{\psi^\mathrm{L}_+} \\ \bra{\psi^\mathrm{L}_-} \\ \end{bmatrix} = \frac{\sqrt{2}}{\varepsilon_+ - \varepsilon_-} \begin{bmatrix} -1 & -\varepsilon_+ \\ +1 & +\varepsilon_- \\ \end{bmatrix} \,, \end{align*}\]where their normalization factors are arranged to fulfill
\[\braket{\psi^\mathrm{R}_\pm | \psi^\mathrm{R}_\pm} = 1, \quad \braket{\psi^\mathrm{L}_n | \psi^\mathrm{R}_m} = \delta_{nm} \,.\]The exceptional point occurs at the branch point of the square root $\varepsilon_+ - \varepsilon_- = 2\sqrt{1-\kappa^2}$ where $\kappa = 1$ and $\varepsilon_+ = \varepsilon_-$. At this exceptional point $\varepsilon_\mathrm{EP} \equiv - \mathrm{i}$, the right wavefunctions coalesce, while the left wavefunctions diverge, rendering $\mathcal{H}_\mathrm{eff}$ non-diagonalizable.
However, since the biorthogonal wavefunctions are exact and symbolic, we can still use them to compute any Hermitian observable $\mathcal{O} = [[O_{11}, O_{12}], [O_{12}^*, O_{22}]]$ via Equation (5) in our Letter:
\[\begin{align*} \braket{O} = \operatorname{Im} \operatorname{Tr} [ \mathcal{O} f_\mathrm{eff}(\mathcal{H}_\mathrm{eff})] = 2 \operatorname{Re}(O_{12}) \operatorname{Im}\left[\frac{f_\mathrm{eff} (\varepsilon_+)-f_\mathrm{eff} (\varepsilon_-)}{\varepsilon_+-\varepsilon_-}\right] + \frac{O_{11}+O_{22}}{2} \,, \end{align*}\]which is associated with the difference quotient of $f_\mathrm{eff}$. Since the $f_\mathrm{eff}$ is analytic in the lower half of the complex plane, its derivative at the exceptional point $\varepsilon_\mathrm{EP}$ exists and thus
\[\begin{align*} \braket{O}_\mathrm{EP} = 2 \operatorname{Re}(O_{12}) \operatorname{Im} [f'_\mathrm{eff} (\varepsilon_\mathrm{EP})] + \frac{O_{11}+O_{22}}{2} \,. \end{align*}\]which is exact and continuous. Therefore, similar to $\sin (x) / x$ that has a removable singularity at $x = 0$, the exceptional point is also a removable singularity for a physical observable $\braket{O}$, whose value is associated with the derivative of the non-Hermitian Fermi-Dirac distribution $f_\mathrm{eff}$.
The above symbolic calculations are based on a toy model. For the non-Hermitian SNS junctions and normal rings studied in our Letter, we append more general discussion on the continuity of the persistent current in Section II-D Current continuity at EPs of the Supplemental Material. We also supplement a Mathematica notebook in our GitHub repository to explicitly show how to analytically derive the limit at the exceptional point.2
-
P.-X. Shen, Z. Lu, J. L. Lado, and M. Trif, Non-Hermitian Fermi-Dirac Distribution in Persistent Current Transport, Physical Review Letters 133, 086301 (2024). ↩
-
GitHub Repository: Non-Hermitian Fermi-Dirac Distribution in Persistent Current Transport. ↩
